The forces that bind protons and neutrons in an atomic nucleus are so strong that when they break, the result is a nuclear explosion. This strong bond is needed to overcome the huge electric repulsion between same charge protons, so the force that holds the nucleus together was called the strong force. It has the odd property that it is zero at short range but increases with distance, an effect analogous to stretching a rubber band. It exchanges no energy, so it isn’t electromagnetic, and increases with distance, so it isn’t gravity. The standard model needed a new force to explain how the atomic nucleus held together.
Its answer was quantum chromo-dynamics (QCD), a field theory derived from quantum electro-dynamics (QED), which explains electro-magnetism as perturbations caused when an electro-magnetic field absorbs or emits photons, as shown in Feynman diagrams. Aiming to repeat the success of QED, QCD proposed a new strong field that emitted gluon particles with a color charge.
In essence, strong field gluons acted to cause effects just as electro-magnetic field photons did, but with three charges (red, blue, and green) that cancel to white, instead of two positive and negative charges that cancel to neutral. Three colors needed anti-colors to work, so to turn a red quark to blue needs an anti-red gluon as well as a blue gluon. It was tricky but the calculations were made to work, and in 1978 the PLUTO project managed to interpret a three-jet Upsilon event in gluon terms. Gluons then joined the standard model particle pantheon, but no-one wondered why a universal field through all space existed for an effect that applied only to quarks.
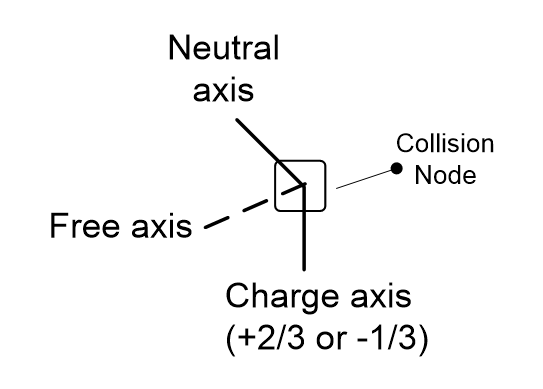
A processing model approaches the same facts differently, based on reverse engineering rather than a field that fits the facts. The quark structure in Figure 4.11 has free photons in one axis that could insert themselves into another quark nearby in a plasma. In Figure 4.12, an extreme photon head is in one quark and its tail in another, so when quarks are side-by-side, they could connect like this.
.
.
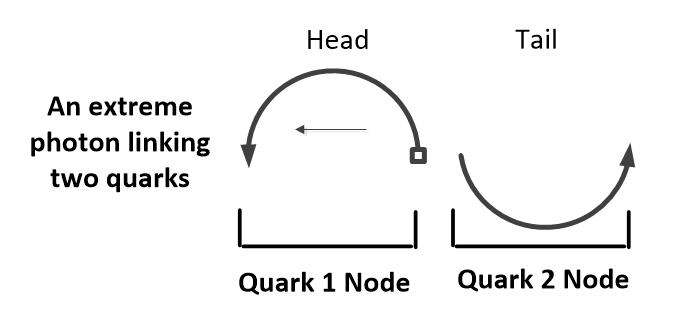
The result is a bond that is initially zero but increases with distance, since as the linked quarks separate, the shared photon wavelength increases to release the energy needed to pull them back together. The further apart the quarks are, the stronger the effect, so side-by-side quarks experience no force unless they try to move apart. Shared photons could then be the elastic bands that bind quarks in the nucleus not gluons from a strong field, but how then do protons and neutrons form?