Lambert’s cosine law is that the intensity of light hitting a surface varies by the cosine of the angle it hits at, so light at right angles to a surface gives all its intensity, but light parallel to it gives none. In angle terms, light at right angles is 90º which has a cosine of one, and parallel light is 0º which has a cosine of zero, so the formula works. In general, the cosine of the angle gives the intensity, so light projects its intensity onto a plane according to angle.
A processing model explains this law in channel terms. A ray of light can use all the channels of a point on its axis, so it has full intensity on that axis but on other channels, its strength projects according to angle following the cosine law. Rays of light on the same axis then compete for the same channels, while rays at right angles don’t compete for channels. In-between, rays share channels based on the cosine of the angle between them, so the cosine law explains how they fill the channels of a point.
In the last section, electrons arose when two extreme light rays overloaded the channels of a line through a point, so can a collision of three extreme rays produce the same effect for a plane? Can the logic applied to a line for electrons be extended to a plane for quarks?
For electrons, the channel capacity of a line was taken to be one, so if light at right angles uses different channels, the bandwidth of a plane through a point is two. Hence, if two extreme light rays fill the channels of a line through a point, four extreme light rays are needed to fill a plane though it.
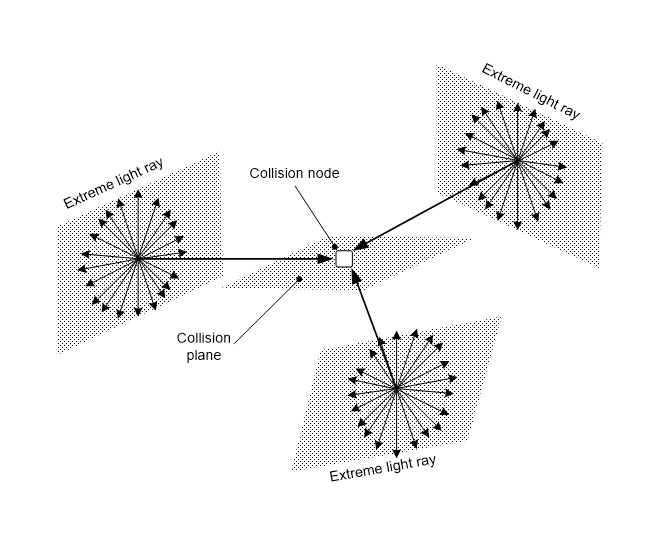
In Figure 4.9, three equal-angle extreme rays meeting at a point aren’t enough to fill the bandwidth of a plane. Each ray fills half the bandwidth of a line axis, but three times a half is 1.5 not two, so the result isn’t stable because there are unfilled channels that another entity could exploit. Dividing the plane bandwidth of two between three axes gives each a two-thirds bandwidth, as three times two-thirds gives the plane bandwidth of two
However, a result that fills some axes completely would be semi-stable, as quarks are, so could a three-way interaction fill two axes, even if the third is unfilled?