The last chapter concluded that our space is a surface within a higher dimensional network, so light can travel in empty space by vibrating at right angles to it. Light then travels on the surface of our space as waves travel on the surface of water.
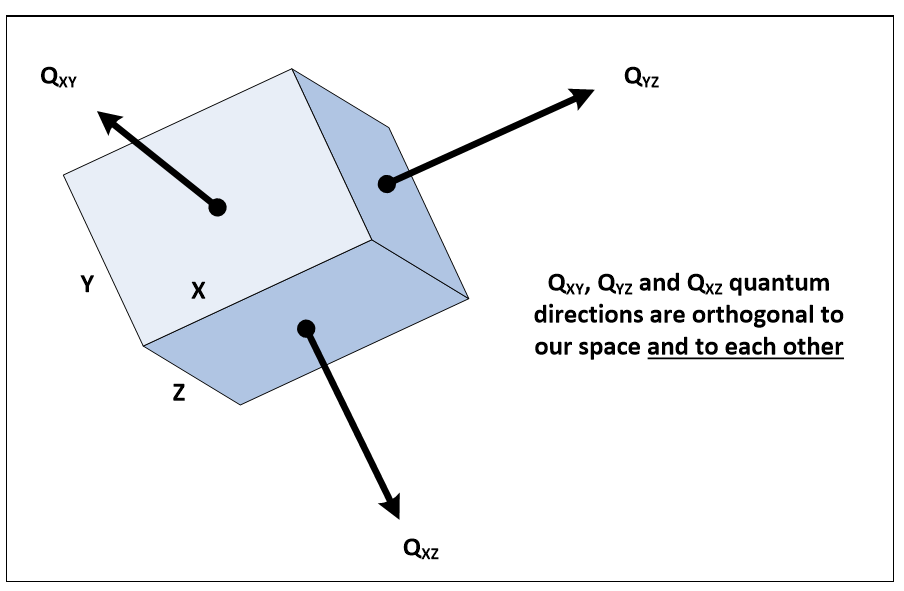
One might suppose that adding a dimension to a three-dimensional space adds one new direction but in mathematics, the result is three new directions at right angles to each other. In Figure 3.18, each of the three planes that cut through a point in our space has a quantum direction outside our space, and they are all at right angles to each other. Adding a dimension outside our space allows three new orthogonal quantum directions (Note 1).
Light as a transverse wave vibrates at right angles to its polarization plane. Current physics calls this direction imaginary, but quantum realism allows three real quantum directions outside our space. Figure 3.18 then suggests that light passing through a point can vibrate in three orthogonal quantum directions, one for each of the three polarization planes it can have.
However, light moving in a given direction has only two independent quantum directions, as its axis of movement uses up one dimension. Hence light can polarize in two independent ways, called vertical and horizontal, as two independent planes cut its axis of movement. The quantum directions of these polarization planes are at right angles to each other, so a filter blocking vertical polarized light doesn’t block horizontal polarized light, and vice-versa.
A filter that blocks vertically polarized light by stopping vibration in one quantum direction doesn’t block the other quantum direction, so horizontally polarized light can pass right through it. Light can vibrate in two orthogonal quantum directions, so what blocks one vibration doesn’t block the other. What then happens when a polarization filter is turned on an angle?
Note 1: If physical space has dimensions (X, Y, Z), let quantum space have dimensions (X, Y, Z, Q), where Q is a fourth quantum dimension. A point in physical space with three orthogonal planes XY, XZ and YZ through it then has three orthogonal quantum directions outside our space. A photon with any polarization plane can vibrate into a quantum direction at right angles to it.